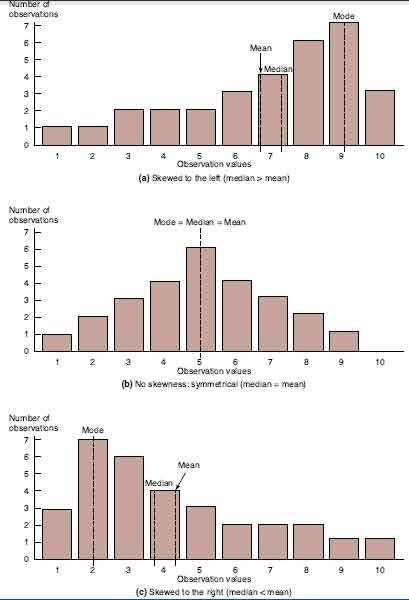
The mean, median, and mode are all useful measures of central tendency, but their value can be limited by unique characteristics of the underlying data. A comparison across alternate measures is useful for determining the extent to which a consistent pattern of central tendency emerges. If the mean, median, and mode all coincide at a single sample observation, the sample data are said to be symmetrical. If the data are perfectly symmetrical, then the distribution of data above the mean is a perfect mirror image of the data distribution below the mean. A perfectly symmetrical distribution is illustrated in Figure. Whereas a symmetrical distribution implies balance in sample dispersion, skewness implies a lack of balance. If the greater bulk of sample observations are found to the left of the sample mean, then the sample is said to be skewed downward or to the left as in Figure. If the greater bulk of sample observations are found to the right of the mean, then the sample is said to be skewed upward or to the right as in Figure). When alternate measures of central tendency converge on a single value or narrow range of values, managers can be confident that an important characteristic of a fairly homogeneous sample of observations has been discovered. When alternate measures of central tendency fail to converge on a single value or range of values, then it is likely that underlying data comprise a heterogeneous sample of observations with important subsample differences. A comparison of alternate measures of central tendency is usually an important first step to determining whether a more detailed analysis of subsample differences is necessary.
The Mean, Median, and Mode
One thought on “Comparative analysis of all measures of central Tendency”