Equi-Marginal Principle (also known as the principle of equal marginal utility or the law of equi-marginal utility) is a fundamental concept in economics that helps individuals and businesses maximize satisfaction or profit. According to this principle, resources should be allocated in such a way that the marginal utility or marginal returns from each resource are equal across all possible uses.
In other words, whether a consumer is trying to maximize their utility or a firm is trying to maximize profit, they will distribute their limited resources (money, labor, time, etc.) among various alternatives so that the additional (marginal) benefit derived from the last unit of resource used in each alternative is equal.
Key Elements of the Equi-Marginal Principle:
- Marginal Utility:
Marginal utility refers to the additional satisfaction or benefit that a person receives from consuming an additional unit of a good or service. As more of a good is consumed, the marginal utility usually decreases, a concept known as diminishing marginal utility.
-
Marginal Productivity/Returns:
In business, marginal productivity or marginal returns refer to the additional output that can be obtained by using an additional unit of input. Like marginal utility, marginal returns also generally diminish as more units of input are added.
- Optimization:
The equi-marginal principle is about optimization. Consumers aim to allocate their resources (income) in such a way that the marginal utility per unit of money spent is equal for all goods. Similarly, firms allocate inputs like labor and capital to maximize profit, ensuring that the marginal returns from each input are equal across all uses.
Formula for the Equi-Marginal Principle
For consumers: The formula for maximizing utility using the equi-marginal principle is as follows:

Example: Allocation of Consumer Budget
Let’s assume a consumer has a budget of $100 to spend on two goods, A and B. The consumer’s goal is to allocate their budget in such a way that the total utility derived from consuming both goods is maximized.
Table of Marginal Utility and Price:
| Units Consumed | Marginal Utility of A (MUA) | Price of A (PA) | MUA/PA | Marginal Utility of B (MUB) | Price of B (PB) | MUB/PB |
| 1 | 20 | $10 | 2 | 24 | $8 | 3 |
| 2 | 18 | $10 | 1.8 | 20 | $8 | 2.5 |
| 3 | 16 | $10 | 1.6 | 16 | $8 | 2 |
| 4 | 14 | $10 | 1.4 | 12 | $8 | 1.5 |
| 5 | 12 | $10 | 1.2 | 8 | $8 | 1 |
From the table, we can see the marginal utility per dollar spent on each good for various levels of consumption.
Allocation Process:
- Initially, the consumer will compare the MU/P ratios for both goods.
- The consumer will spend their first dollar on Good B because it provides a higher marginal utility per dollar (3) than Good A (2).
- After consuming the first unit of Good B, the consumer will compare the MU/P ratios again. Since MUB/PB=2.5 is still higher than MUA/PA=2, the consumer will purchase another unit of Good B.
- This process will continue until the MU/P ratios for both goods are equal or the consumer’s budget is exhausted.
In this case, the consumer might end up purchasing 2 units of Good A and 3 units of Good B, at which point the marginal utility per dollar for both goods becomes approximately equal, maximizing their total utility.
Example: Firm’s Input Allocation
Let’s assume a firm has two inputs: labor (L) and capital (K). The firm wants to allocate these inputs to maximize profit, with the marginal product and cost data as follows:
| Input | Marginal Product of Labor (MPL) | Cost of Labor (CL) | MPL/CL | Marginal Product of Capital (MPK) | Cost of Capital (CK) | MPK/CK |
| 1 | 50 | $10 | 5 | 80 | $20 | 4 |
| 2 | 40 | $10 | 4 | 70 | $20 | 3.5 |
| 3 | 30 | $10 | 3 | 60 | $20 | 3 |
| 4 | 20 | $10 | 2 | 50 | $20 | 2.5 |
| 5 | 10 | $10 | 1 | 40 | $20 | 2 |
The firm’s goal is to allocate labor and capital in such a way that the marginal product per unit of cost is equal for both inputs.
Allocation Process:
- Initially, the firm compares the MP/C ratios for labor and capital.
- The firm will allocate its first dollar towards labor, where MPL/CL=5 is greater than MPK/CK=4.
- After allocating more resources, the firm will continue comparing the ratios.
- The firm will keep allocating resources until the marginal product per unit cost for both labor and capital is equal.
In this case, the optimal allocation would involve using 2 units of labor and 1 unit of capital, where the marginal products per unit cost are equal (4), maximizing the firm’s profit.
Importance of the Equi-Marginal Principle:
- Efficient Allocation:
The equi-marginal principle ensures the efficient allocation of resources, whether for consumers aiming to maximize utility or firms aiming to maximize profit. By allocating resources where they provide the highest marginal benefit, both individuals and businesses can make the best possible use of their limited resources.
-
Economic Decision-Making:
This principle is a key component of rational decision-making in economics. It helps in determining the optimal quantity of goods to consume, the best mix of inputs to use in production, or even the best way to allocate time among different activities.
- Flexibility:
The equi-marginal principle can be applied across various fields of economics, from consumer theory and production theory to cost minimization and utility maximization.
Explanation of the Law:
In order to get maximum satisfaction out of the funds we have, we carefully weigh the satisfaction obtained from each rupee ‘had we spend If we find that a rupee spent in one direction has greater utility than in another, we shall go on spending money on the former commodity, till the satisfaction derived from the last rupee spent in the two cases is equal.
It other words, we substitute some units of the commodity of greater utility tor some units of the commodity of less utility. The result of this substitution will be that the marginal utility of the former will fall and that of the latter will rise, till the two marginal utilities are equalized. That is why the law is also called the Law of Substitution or the Law of equimarginal Utility.
Suppose apples and oranges are the two commodities to be purchased. Suppose further that we have got seven rupees to spend. Let us spend three rupees on oranges and four rupees on apples. What is the result? The utility of the 3rd unit of oranges is 6 and that of the 4th unit of apples is 2. As the marginal utility of oranges is higher, we should buy more of oranges and less of apples. Let us substitute one orange for one apple so that we buy four oranges and three apples.
Now the marginal utility of both oranges and apples is the same, i.e., 4. This arrangement yields maximum satisfaction. The total utility of 4 oranges would be 10 + 8 + 6 + 4 = 28 and of three apples 8 + 6 + 4= 18 which gives us a total utility of 46. The satisfaction given by 4 oranges and 3 apples at one rupee each is greater than could be obtained by any other combination of apples and oranges. In no other case does this utility amount to 46. We may take some other combinations and see.
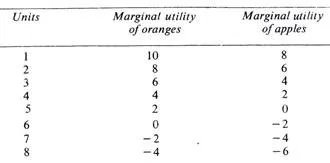
We thus come to the conclusion that we obtain maximum satisfaction when we equalize marginal utilities by substituting some units of the more useful for the less useful commodity. We can illustrate this principle with the help of a diagram.
Diagrammatic Representation:
In the two figures given below, OX and OY are the two axes. On X-axis OX are represented the units of money and on the Y-axis marginal utilities. Suppose a person has 7 rupees to spend on apples and oranges whose diminishing marginal utilities are shown by the two curves AP and OR respectively.
The consumer will gain maximum satisfaction if he spends OM money (3 rupees) on apples and OM’ money (4 rupees) on oranges because in this situation the marginal utilities of the two are equal (PM = P’M’). Any other combination will give less total satisfaction.
Let the purchase spend MN money (one rupee) more on apples and the same amount of money, N’M'( = MN) less on oranges. The diagram shows a loss of utility represented by the shaded area LN’M’P’ and a gain of PMNE utility. As MN = N’M’ and PM=P’M’, it is proved that the area LN’M’P’ (loss of utility from reduced consumption of oranges) is bigger than PMNE (gain of utility from increased consumption of apples). Hence the total utility of this new combination is less.

We then, conclude that no other combination of apples and oranges gives as great a satisfaction to the consumer as when PM = P’M’, i.e., where the marginal utilities of apples and oranges purchased are equal, with given amour, of money at our disposal.
Limitations of the Law of Equi-marginal Utility
Like other economic laws, the law of equimarginal utility too has certain limitations or exceptions. The following are the main exception.
(i) Ignorance
If the consumer is ignorant or blindly follows custom or fashion, he will make a wrong use of money. On account of his ignorance he may not know where the utility is greater and where less. Thus, ignorance may prevent him from making a rational use of money. Hence, his satisfaction may not be the maximum, because the marginal utilities from his expenditure cannot be equalised due to ignorance.
(ii) Inefficient Organisation
In the same manner, an incompetent organiser of business will fail to achieve the best results from the units of land, labour and capital that he employs. This is so because he may not be able to divert expenditure to more profitable channels from the less profitable ones.
(iii) Unlimited Resources
The law has obviously no place where this resources are unlimited, as for example, is the case with the free gifts of nature. In such cases, there is no need of diverting expenditure from one direction to another.
(iv) Hold of Custom and Fashion
A consumer may be in the strong clutches of custom, or is inclined to be a slave of fashion. In that case, he will not be able to derive maximum satisfaction out of his expenditure, because he cannot give up the consumption of such commodities. This is specially true of the conventional necessaries like dress or when a man is addicted to some intoxicant.
(v) Frequent Changes in Prices
Frequent changes in prices of different goods render the observance of the law very difficult. The consumer may not be able to make the necessary adjustments in his expenditure in a constantly changing price situation.
2 thoughts on “Equi Marginal Utility”