Poisson Distribution: Importance Conditions Constants, Fitting of Poisson Distribution

Poisson distribution is a probability distribution used to model the number of events occurring within a fixed interval of time, space, or other dimensions, given that these events occur independently and at a constant average rate.

Importance

1. Modeling Rare Events: Used to model the probability of rare events, such as accidents, machine failures, or phone call arrivals.
2. Applications in Various Fields: Applicable in business, biology, telecommunications, and reliability engineering.
3. Simplifies Complex Processes: Helps analyze situations with numerous trials and low probability of success per trial.
4. Foundation for Queuing Theory: Forms the basis for queuing models used in service and manufacturing industries.
5. Approximation of Binomial Distribution: When the number of trials is large, and the probability of success is small, Poisson distribution approximates the binomial distribution.

Conditions for Poisson Distribution

1. Independence: Events must occur independently of each other.
2. Constant Rate: The average rate (λ) of occurrence is constant over time or space.
3. Non-Simultaneous Events: Two events cannot occur simultaneously within the defined interval.
4. Fixed Interval: The observation is within a fixed time, space, or other defined intervals.

Constants

1. Mean (λ): Represents the expected number of events in the interval.
2. Variance (λ): Equal to the mean, reflecting the distribution’s spread.
3. Skewness: The distribution is skewed to the right when λ is small and becomes symmetric as λ increases.
4. Probability Mass Function (PMF): P(X = k) = [e^−λ*λ^k] / k!, Where $k$ is the number of occurrences, $e$ is the base of the natural logarithm, and λ is the mean.

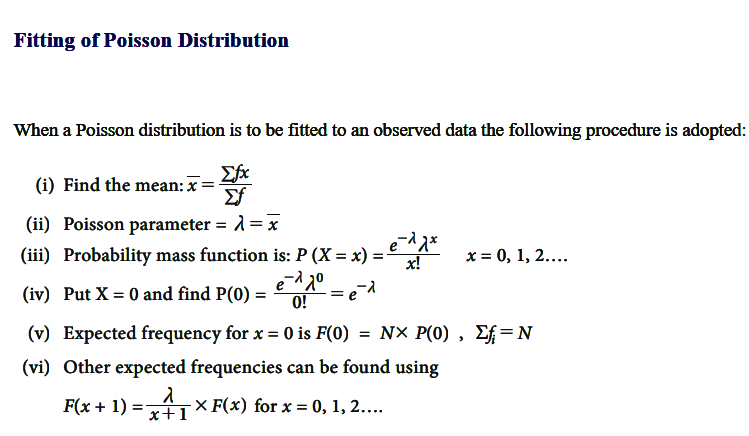
Fitting of Poisson Distribution

When a Poisson distribution is to be fitted to an observed data the following procedure is adopted: